Q.
Light from a discharge tube containing hydrogen atoms falls on a piece of sodium due to the transition of electron from $4^{\text {th }}$ orbit to $2^{\text {nd }}$ orbit. Work function of sodium is $1.83\, eV$. The fastest moving photoelectron is allowed to enter in a magnetic field, which is perpendicular to the direction of motion of photoelectron as shown in the figure. Find the distance (in $\mu m$ ) covered by the electron in the magnetic field. $\left(B=1 T , \pi^{2}=10\right.$, Mass of electron $=9 \times 10^{-31} \,kg$ and $R=$ Radius of the path that the most energetic electron takes in the presence of applied magnetic field)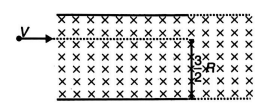
Atoms
Solution: