Question

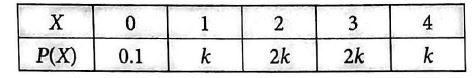
Let $X$ denote the number of hours you study on a Sunday. Also it is known that
$P(X=x) = \begin{cases} 0.1 \quad ,& \text{if } x = 0 \\ kx \quad,& \text{if } x=1 \,\text{or }\, 2 \\ k(5-x) ,& \text{if } x = 3 \,\text{or } \,4 \\ 0 \quad , & \text{otherwise } \end{cases} $
where $k$ is a constant.
What is the probability that you study atleast two hours ?

• A. $0.55$
• B. $0.15$
• C. $0.75$
• D. $0.3$

Answer 1

Answer: (C)

From the given information, we find that the probability distribution of $X$ is

We know that $\sum p_{i} = 1$
$\Rightarrow 0.1 + k + 2k + 2k + k = 1$
$\Rightarrow 6k = 1- 0.1 = 0.9$
$\Rightarrow k=0.15$
Now, $P$(you study atleast two hours) $= P\left(X \ge 2\right)$
$= P\left(2\right) + P\left(3\right) + P\left(4\right) = 2k + 2k + k$
$= 5k = 5 \times 0.15 = 0.75$