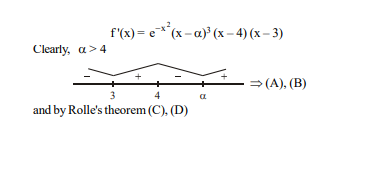
Q. Let $f ( x )=\int\limits_0^{ x } e ^{- t ^2}( t -\alpha)^3\left( t ^2-7 t +12\right) dt$ for all $x \in(0, \infty)$, if $f$ has a local maximum at $x =$ 4 and a local minimum at $x=3$ and $\alpha \in[0,10]$, then
Application of Derivatives
Solution:
Correct answer is (a) number of possible integral value of $\alpha$ is 6 .Correct answer is (b) $x =\alpha$ is a point of local minimum.Correct answer is (c) there exists atleast two $c_1, c_2 \in(0, \infty)$ such that $f^{\prime \prime}\left(c_1\right)=0$ and $f^{\prime \prime}\left(c_2\right)=0$.Correct answer is (d) there exists some $c \in(0, \infty)$ such that $f^{\prime \prime \prime}(c)=0$.