Q. Let ex−e−xex+e−x=ln√1+x1−x , then find x .
NTA AbhyasNTA Abhyas 2022
Solution:
Let f(x)=ex−e−xex+e−x
⇒y=ex−e−xex+e−x
⇒y=e2x−1e2x+1
⇒e2xy+y=e2x−1
e2x(y−1)=−1−y
e2x=1+y1−y
ex=√1+y1−y
x=ln√1+y1−y
f−1(y)=ln√1+y1−y
f−1(x)=ln√1+x1−x
Now, given equation is
ex−e−xex+e−x=ln√1+x1−x
⇒f(x)=f−1(x)
The solution of f(x)=f−1(x) is always obtained on y=x line
⇒ln√1+x1−x=x
⇒e2x=1+x1−x
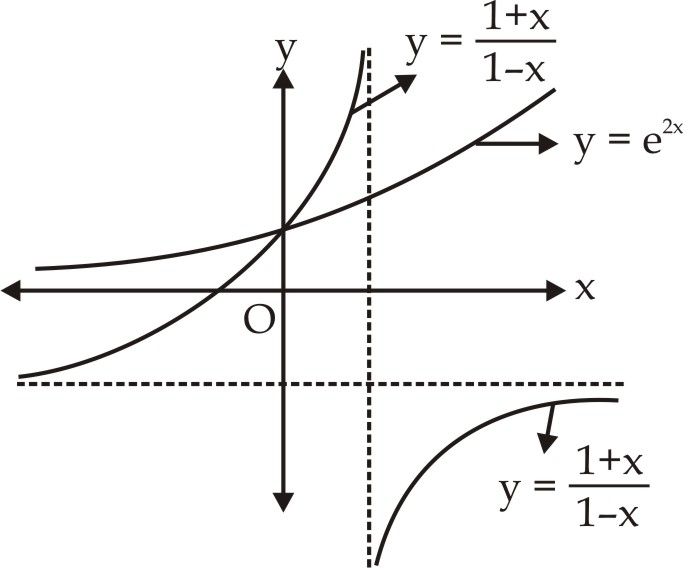
Only one solution x=0 .
⇒y=ex−e−xex+e−x
⇒y=e2x−1e2x+1
⇒e2xy+y=e2x−1
e2x(y−1)=−1−y
e2x=1+y1−y
ex=√1+y1−y
x=ln√1+y1−y
f−1(y)=ln√1+y1−y
f−1(x)=ln√1+x1−x
ex−e−xex+e−x=ln√1+x1−x
⇒f(x)=f−1(x)
The solution of f(x)=f−1(x) is always obtained on y=x line
⇒ln√1+x1−x=x
⇒e2x=1+x1−x

Only one solution x=0 .