Q.
In the Wheat stone's network given, $ P=10\Omega , $ $ Q=20\Omega ,R=15\Omega , $ $ S=30\Omega , $ the current passing through the battery (of negligible internal resistance) is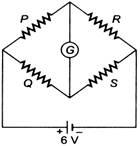
MGIMS WardhaMGIMS Wardha 2008
Solution: