Question

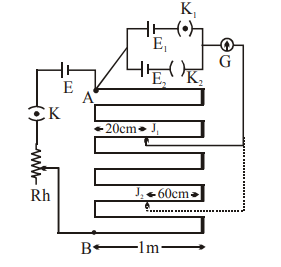
In the given circuit of potentiometer, the potential difference $E$ across $A B$ (10m length) is larger than $E _{1}$ and $E _{2}$ as well. For key $K _{1}$ (closed), the jockey is adjusted to touch the wire at point $J_{1}$ so that there is no deflection in the galvanometer. Now the first battery $\left( E _{1}\right)$ is replaced by second battery $\left( E _{2}\right)$ for working by making $K _{1}$ open and $K _{2}$ closed. The galvanometer gives then null deflection at $J _{2}$. The value of $\frac{E_{1}}{E_{2}}$ is $\frac{a}{b},$ where a $=$___

Answer 1

Length of $AB =10 m$
For battery $E _{1}$, balancing length is $l_{1}$
$l_{1}=380 cm [$ from end $A ]$
For battery $E _{2}$, balancing length is $l_{2}$
$l_{2}=760 cm [$ from end $A ]$
Now, we know that $\frac{ E _{1}}{ E _{2}}=\frac{l_{1}}{l_{2}}$
$\Rightarrow \frac{E_{1}}{E_{2}}=\frac{380}{760}=\frac{1}{2}=\frac{a}{b}$
$\therefore a=1 \& b=2$
$a =1$