Q.
In the figure shown, the coefficient of static friction between the blocks is $0.1$, while the floor is frictionless. Find the minimum value of force $F$ (in $N$ ) to cause sliding between the blocks $A$ and $B$ of masses $5 \,kg$ and $10 \,kg$, respectively, when the angle of string is $53^{\circ}$ with horizontal. (Use $\sin 53^{\circ}=4 / 5$ and $\cos 53^{\circ}=3 / 5$ )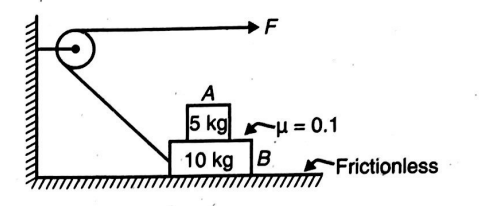
Laws of Motion
Solution:
