Q.
In the figure shown, a lift goes downward with a constant retardation. An observer in the lift observes a simple pendulum with time period $2\, s$. The length of the string which is used to suspend the ball from the ceiling is $2.0\, m$. If the retardation of the lift is $\alpha m / s ^{2}$ then find the value of $\frac{\alpha}{2}$.
[use: $\pi^{2}=10$ and $g=10\, m / s ^{2}$ ]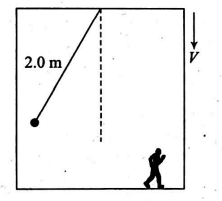
Oscillations
Solution: