Question

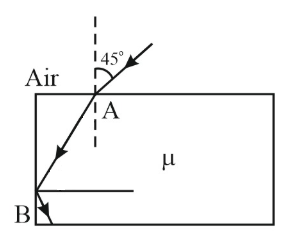
In the figure given below, for an angle of incidence $45^\circ $ at the top surface, what is the minimum refractive index needed for total internal reflection at the vertical face?

• A. $\frac{\sqrt{2} + 1}{2}$
• B. $\sqrt{\frac{1}{2}}$
• C. $\sqrt{\frac{3}{2}}$
• D. $\sqrt{2}+1$

Answer 1

Answer: (C)

At point $A$ , by Snell's law

$\mu=\frac{\sin 45^{\circ}}{\sin r} \Rightarrow \sin r=\frac{1}{\mu \sqrt{2}} \ldots$ (i)
At point $B$, total internal reflection with $\sin i_{1}=\frac{1}{\mu}$
From figure, $i_{1}=90^{\circ}-r \ldots$ (ii)
$\sin \left(90^{\circ}-r\right)=\frac{1}{\mu} \Rightarrow \cos r=\frac{1}{\mu} \ldots .$ (iii)
Now, $\cos r=\sqrt{1-\sin ^{2} r}=\sqrt{1-\frac{1}{2 \mu^{2}}}=\sqrt{\frac{2 \mu^{2}-1}{\mu^{2}}}$
From equation (ii) and (iii), $\frac{1}{\mu}=\sqrt{\frac{2 \mu^{2}-1}{2 \mu^{2}}}$
Squaring both sides and then solving, we get
$\mu=\sqrt{\frac{3}{2}}$