Q.
In the figure given below, a block of mass $M=490 g$ placed on a frictionless table is connected with two springs having same spring constant $\left( K =2\, N m ^{-1}\right)$. If the block is horizontally displaced through ' $X$ ' $m$ then the number of complete oscillations it will make in $14 \pi$ seconds will be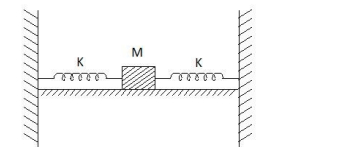
Solution:
