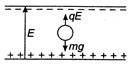
Question

In Millikans oil drop experiment, an oil drop of radius $r$ and charge $q$ is held in equilibrium between plates of a parallel plate capacitor when the potential difference is $V$. To keep a drop of radius $2 r$ with charge $2q$ in equilibrium between the plates, the potential difference required is :

• A. V
• B. 2 V
• C. 4 V
• D. 8 V

Answer 1

Answer: (C)

In Millikans oil drop experiment, in order that the drop stays stationary force due to electric field should equal to gravitational force.
$\therefore F=q E=m g \ldots$ (i)
Also, mass $=$ volume $\times$ density
$m=\frac{4}{3} \pi r^{3} \rho$ and $E=\frac{V}{d}$
Hence, Eq. (i), becomes
$\frac{4}{3} \pi r^{3} \rho g=q \cdot \frac{V}{d} $
$\therefore \frac{V_{1}}{V_{2}}=\left(\frac{r_{1}}{r_{2}}\right)^{3}\left(\frac{q_{2}}{q_{1}}\right)$
Given, $r_{2}=2 r, r_{1}=r, q_{2}=2 q, q_{1}=q$
$ \therefore \frac{V_{1}}{V_{2}}=\left(\frac{r}{2 r}\right)^{3}\left(\frac{q_{2}}{q_{1}}\right)$
$ \therefore V_{2}=4 V_{1}=4 V(\therefore V_{1}=V$ (given))