1. Tardigrade
  2. Question
  3. Mathematics
  4. If z be a complex number satisfying |Re(z)|+|Im(z)|=4, then |z| cannot be :

Question Error Report

Thank you for reporting, we will resolve it shortly

Back to Question

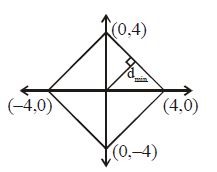
Q. If $z$ be a complex number satisfying $\left|Re\left(z\right)\right|+\left|Im\left(z\right)\right|=4,$ then $|z|$ cannot be :

JEE MainJEE Main 2020Complex Numbers and Quadratic Equations

Solution:

$z = x + iy \quad\quad|x| + |y| = 4$
$\left|z\right| = \sqrt{x^{2}+y^{2}} \Rightarrow \left|z\right|_{min} = \sqrt{8} \,\& \,\left|z\right|_{max} = 4 = \sqrt{16}$
So |z| cannot be $\sqrt{7}$

Solution Image