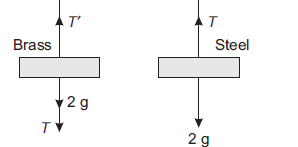
Q.
If the ratio of lengths, radii and Young's modulus of steel and brass wires shown in the figure are $a, b$ and $c$ respectively, the ratio between the increase in lengths of brass and steel wires would be
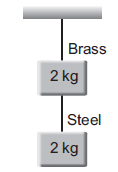
EAMCETEAMCET 2009
Solution: