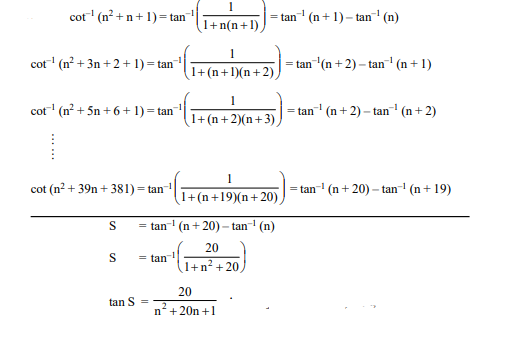
Q. If $S=\cot ^{-1}\left(n^2+n+1\right)+\cot ^{-1}\left(n^2+3 n+3\right)+\cot ^{-1}\left(n^2+5 n+7\right)+\ldots \ldots \ldots+\cot ^{-1}\left(n^2+39 n+381\right)$, then $\tan S$ is equal to
Inverse Trigonometric Functions
Solution:
Correct answer is (a) $\frac{20}{n^2+20 n+1}$