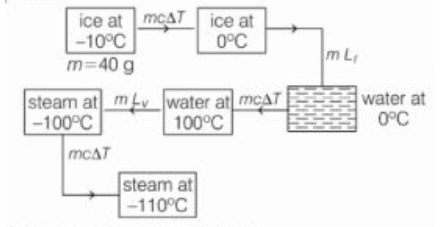
Q.
How much thermal energy is required to change a $40\, g$ ice cube from solid at $-10^{\circ} C$ to steam at $110^{\circ} C$.
[Assume latent heat of fusion for water $=80 \,kcal / kg$, specific heat of water $=1\, k\,cal / kg { }^{\circ} C$,
specific heat of Ice $=0.5\, k\,cal kg { }^{\circ} C$,
specific heat of steam $=0.48\, k\,cal / kg ^{\circ} C$,
latent heat of vaporisation of water- $540 \,kcal / kg { }^{\circ} C$ ]
TS EAMCET 2020
Solution: