Q.
Helium gas goes through a cycle $A B C D A$ (consisting of two isochoric and isobaric lines) as shown in figure. Find efficiency of this cycle. (Assume the gas to be close to an ideal gas)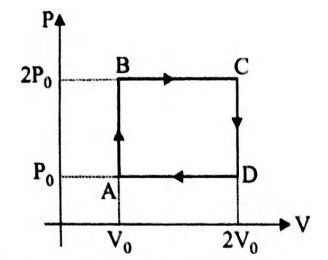
Thermodynamics
Solution:
