Question

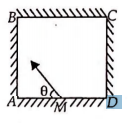
Four identical mirrors are made to stand vertically to form a square arrangement as shown in a top view. A ray starts from the midpoint $M$ of mirror $AD$ and after two reflections reaches corner $D$. Then, angle $\theta$ must be

• A. $tan^{-1}\left(0.75\right)$
• B. $cot^{-1}\left(0.75\right)$
• C. $sin^{-1}\left(0.75\right)$
• D. $cos^{-1}\left(0.75\right)$

Answer 1

Answer: (B)

The ray starting from point $M$ at an angle $\theta$ reaches the corner $D$ at the right along a parallel path. Let $a$ be the length of the side.

From figure,
$tan\, \theta = \frac{x}{\left(a/2\right)}\quad...\left(i\right) $
$tan \,\theta = \frac{a-x}{y} \quad...\left(ii\right) $
$ tan\, \theta = \frac{a}{a-y} \quad...\left(iii\right)$
From $ \left(i\right)$ and $\left(ii\right)$, we get
$\frac{2x}{a}= \frac{a-x}{y} $ or $ 2xy = a^{2} -xa \quad...\left(iv\right)$
From $\left(ii\right)$ and $\left(iii\right)$, we get
$ \frac{a-x}{y} = \frac{a}{a-y}$
$ \Rightarrow 3xy = 2ay $ (Using $(iv)$)
$ x = \frac{2a}{3}$
Substituting this value of $x$ in equation $(i)$, we get
$tan \,\theta = \frac{\left(2a/3\right)}{\left(a/2\right)} = \frac{4}{3}$
$ \therefore cot\, \theta = \frac{1}{ tan\, \theta} = \frac{3}{4} $
or $\theta = cot^{-1}\left(0.75\right)$