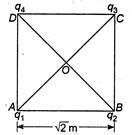
Q. Four charges $ {{q}_{1}}=2\times {{10}^{-8}}C,{{q}_{2}}=-2\times {{10}^{-8}}C, $ $ {{q}_{3}}=-3\times {{10}^{-8}}C $ and $ {{q}_{4}}=6\times {{10}^{-8}}C $ are placed at four comers of a square of side $ \sqrt{2}m $ . What is the potential at the centre of the square?
JamiaJamia 2006
Solution:
The potential at a point r is given by $ V=\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{q}{r} $
$ \therefore $ $ OA=OB=OC=OD=\frac{AC}{2}=\frac{2}{2} $ $ =1 $ Potential at the centre $ V=\frac{1}{4\pi {{\varepsilon }_{0}}}\left( \frac{{{q}_{1}}}{OA}+\frac{{{q}_{2}}}{OB}+\frac{{{q}_{3}}}{OC}+\frac{{{q}_{4}}}{OD} \right) $ $ V=9\times {{10}^{9}} $ $ \times \left( \begin{align} & \frac{2\times {{10}^{8}}}{1}+\frac{-2\times {{10}^{-8}}}{1} \\ & \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,+\frac{-3\times {{10}^{8}}}{1}+\frac{6\times {{10}^{-8}}}{1} \\ \end{align} \right) $