Question

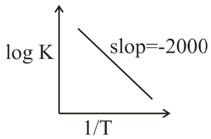
For a reaction, consider the plot of $logK$ versus $\frac{1}{ T}$ given in the figure.
If rate constant of this reaction at $\text{400 K is10}^{- 5}sec^{- 1}$ then the rate constant at $500K$ is :-

• A. $2\times 10^{- 4}sec^{- 1}$
• B. $10^{- 6}sec^{- 1}$
• C. $10^{- 4 }sec^{- 1}$
• D. $4\times 10^{- 4}sec^{- 1}$

Answer 1

Answer: (C)

$E_{a}=2000\times 2.303R$
$log\frac{K_{2}}{ K_{1}}=\frac{E_{a}}{2 . 303 R}\left(\frac{1}{ T_{1}} - \frac{1}{ T_{2}}\right)$
$log\frac{K_{2}}{\left(10\right)^{- 5}}=\frac{2000 \times 2 . 303 R}{2 . 303 R}\left(\frac{1}{400} - \frac{1}{500}\right)$
$log\frac{K_{2}}{10^{- 5}}=2000\times \frac{1}{2000}$
$log\frac{K_{2}}{10^{- 5}}=1$
$\frac{K_{2}}{10^{- 5}}=10\Rightarrow K_{2}=10\times 10^{- 5}$
$=10^{- 4 }sec^{- 1}$