Q.
For a particle executing SHM, the displacement $x$ is given by $ \, x=Acos \omega t$ . Identify the graph which represents the variation of potential energy (PE) as a function of time $t$ and displacement $x$ .
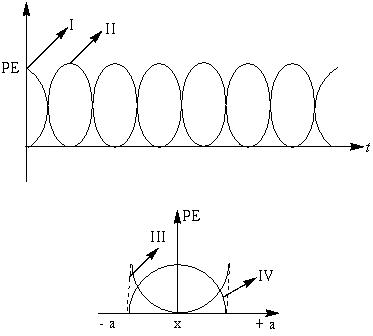
NTA AbhyasNTA Abhyas 2022
Solution: