Q.
Find the electric field vector at $P(b, b, b)$ due to three infinitely long lines of charges along $ x,y $ and z-axes, respectively. The charge density, i.e. charge per unit length of each wire is $ \sigma . $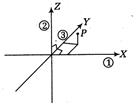
Jharkhand CECEJharkhand CECE 2015
Solution: