Q.
Figure shows two identical particles 1 and 2 , each of mass $m$, moving in opposite directions with same speed $\vec{v}$ along parallel lines. At a particular instant, $\vec{r}_{1}$ and $\vec{r}_{2}$ are their respective position vectors drawn from point $A$ which is in the plane of the parallel lines. Which of the following is the correct statement?
[Here, $\otimes$ represents a unit vector going into the page and $\odot$ represents a unit vector coming out of the page.]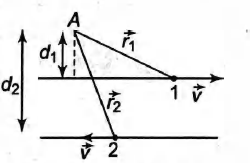
System of Particles and Rotational Motion
Solution: