Q.
Figure shows three spherical and equipotential surfaces A, B and C round a point charge q. The potential difference $ {{V}_{A}}-{{V}_{B}}={{V}_{B}}-{{V}_{C}}. $ If $ {{t}_{1}} $ and $ {{t}_{2}} $ be the distance between them. Then: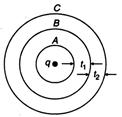
WBJEEWBJEE 2006
Solution: