Q.
Figure below shows three circuits consisting of concentric circular arcs and straight radial lines. The center of the circle is shown by the dot. Same current flows through each of the circuits. If $B_{1}, B_{2}, B_{3}$ are the magnitudes of the magnetic field at the center. Which of the following is true?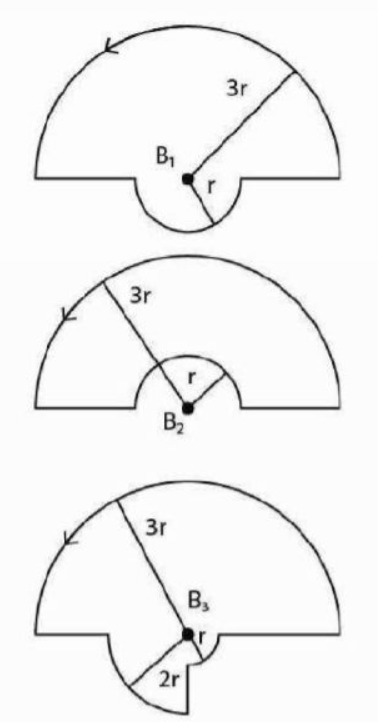
TS EAMCET 2020
Solution:


