Q.
Consider
Minimize $z = 3x + 2y$
subject to $x + y \geq 8 $
$3x + 5y \leq 15$
$x, y \geq 0$
It has :
Linear Programming
Solution:
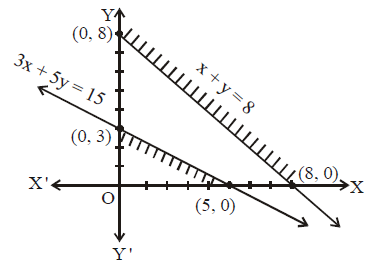
Given problem is minimize z = 3x + 2y subject to x +y $\geq$ 8,
3x + 5y $\leq$ 15
x, y $\geq$ 0
First we convert these inequations into equations and draw the graph.
Since, there is no feasible region, therefore no feasible solution.