Question

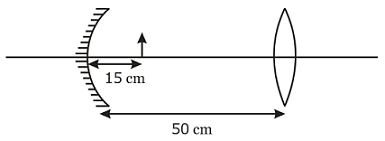
Consider a concave mirror and a convex lens (refractive index = $1.5$ ) of focal length $10$ $cm$ each, separated by a distance of $50$ $cm$ in air (refractive index = $1$ ), as shown in the figure. An object is placed at a distance of $15$ $cm$ from the mirror. Its erect image, formed by this combination, has magnification $M_{1}$ . When the set-up is kept in a medium of refractive index $\frac{7}{6}$ , the magnification becomes $M_{2}$ . The magnitude $\left|\frac{M_{2}}{M_{1}}\right|$ is

• A. 7
• B. 5
• C. 3
• D. 6

Answer 1

Answer: (A)

For reflection from a concave mirror,
$\frac{1}{v}+\frac{1}{u}=\frac{1}{f}\Rightarrow \frac{1}{v}-\frac{1}{15}= \, \frac{- 1}{10}$
$\frac{1}{v}=\frac{1}{15}-\frac{1}{10}=\frac{- 1}{30}$
$\therefore v=-30$
Magnification $\left(m_{1}\right)=-\frac{v}{u}=-2$
Now for refraction from lens,
$\frac{1}{v}-\frac{1}{u}=\frac{1}{f}\Rightarrow \frac{1}{v}=\frac{1}{10}-\frac{1}{20}=\frac{1}{20}$
$\therefore $ Magnification $\left(m_{2}\right)=\frac{v}{u}=-1$
$\therefore M_{1}=m_{1}m_{2}=2 \, $
Now when the set-up is immersed in liquid, no effect for the image formed by mirror.
We have $\left(\mu_{\mathrm{L}}-1\right)\left(\frac{1}{\mathrm{R}_1}-\frac{1}{\mathrm{R}_2}\right)=\frac{1}{10}$
$\Rightarrow \left(\frac{1}{R_{1}} - \frac{1}{R_{2}}\right)=\frac{1}{5}$
When lens is immersed in liquid,
$\frac{1}{\mathrm{f}_{\text {lens }}}=\left(\frac{\mu_{\mathrm{L}}}{\mu_{\mathrm{S}}}-1\right)\left(\frac{1}{\mathrm{R}_1}-\frac{1}{\mathrm{R}_2}\right)=\frac{2}{7} \times \frac{1}{5}=\frac{2}{35}$
$\therefore \frac{1}{v}-\frac{1}{u}=\frac{1}{f_{L i q u i d}}$
$\Rightarrow \frac{1}{v}=\frac{2}{35}-\frac{1}{20}=\frac{8 - 7}{140}=\frac{1}{140}$
$\therefore $ Magnification $=-\frac{140}{20}=-7$
$\therefore M_{2}=2\times 7=14$
$\therefore \left|\frac{M_{2}}{M_{1}}\right|=7$