Question

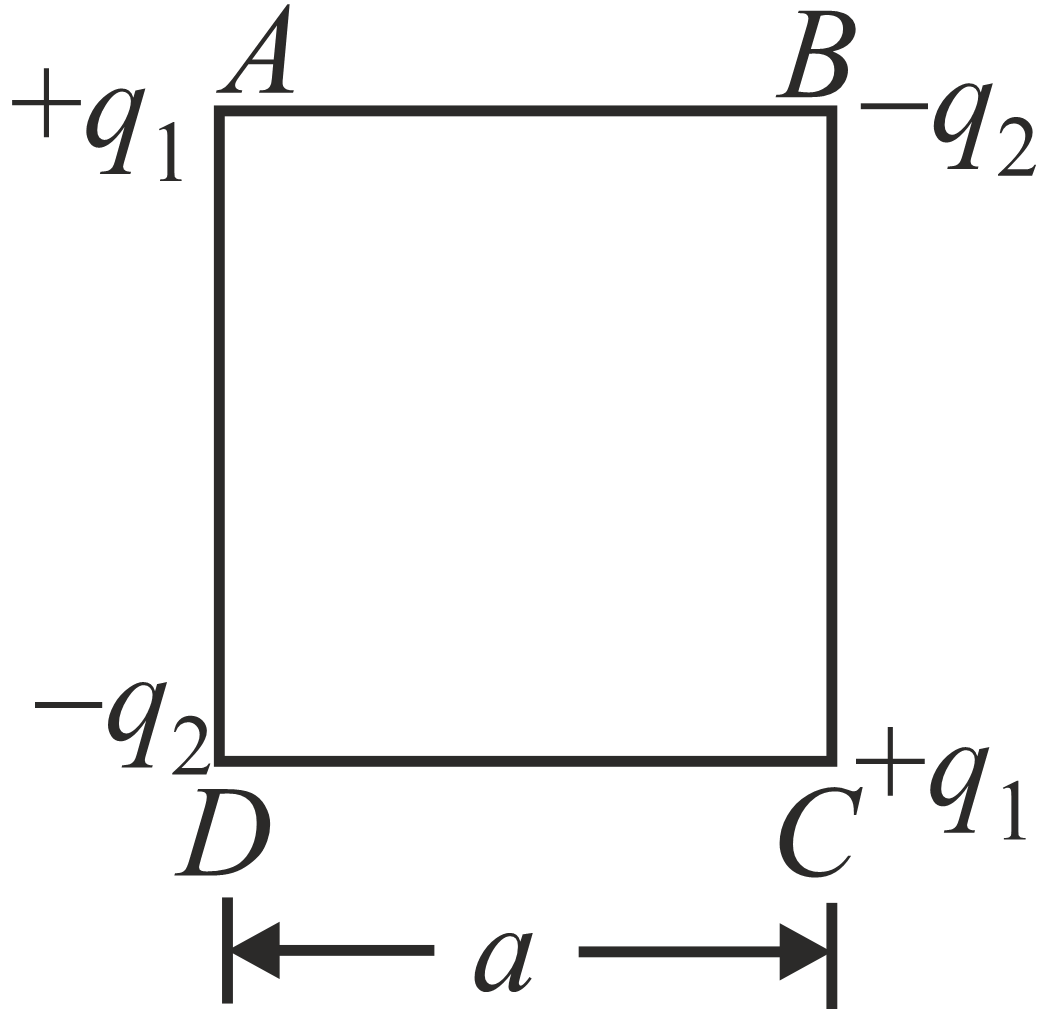
Charges are placed at the corners of a square of side $a$ , as shown in the following figure. The charged particle placed at $A$ is in equilibrium. The ratio $\frac{q_{1}}{q_{2}}$ is

• A. $1$
• B. $2\sqrt{2}$
• C. $\frac{1}{\sqrt{2}}$
• D. $\frac{3}{\sqrt{2}}$

Answer 1

Answer: (B)

The charge at $A$ experiences three forces, $F_{1}$ , $F_{2}$ and $F_{3}$ , as shown in the figure.

Now, $F_{1}=\frac{1}{4 \pi ε_{0} \, }\frac{q_{1} q_{2}}{a^{2}}$ (along $AB$ )
$F_{2}=\frac{1}{4 \pi ε_{0} \, }\frac{q_{1} q_{2}}{a^{2}}$ (along $AD$ )
$F_{3}=\frac{1}{4 \pi ε_{0} \, }\frac{q_{1}^{2}}{2 a^{2}}$
The resultant of $F_{1}$ and $F_{2}$ should be equal and opposite to $F_{3}$ , to keep the system in equilibrium.
Resultant of $F_{1}$ and $F_{2}$ i.e. $F_{R}=\sqrt{F_{1}^{2} + \, F_{2}^{2}}$
$=\frac{1}{4 \pi \, }\frac{q_{1} q_{1}}{\epsilon _{0} a^{2}}\sqrt{2}$
For equilibrium,
$\frac{1}{4 \pi ε_{0}}\frac{q_{1} q_{2}}{a^{2}}\sqrt{2}=\frac{1}{4 \pi ε_{0}}\frac{q_{1}^{2}}{2 a^{2}}$
$\frac{q_{1}}{q_{2}}=2\sqrt{2}$