Q.
Block $ A $ of mass $ m $ and block $ B $ of mass $ 2m $ are placed on a fixed triangular wedge by means of a massless, inextensible string and a frictionless pulley as shown in figure. The wedge is inclined at $ 45^{\circ} $ to the horizontal on both the sides. If the coefficient of friction between the block $ A $ and the wedge is $ 2/3 $ and that between the block $ B $ and the wedge is $ 1/3 $ and both the blocks $ A $ and $ B $ are released from rest, the acceleration of $ A $ will be
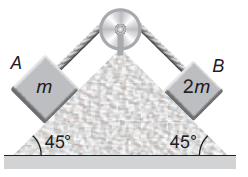
Solution:
