Question

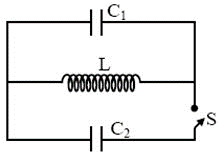
At the moment $t=0$ , when the charge on the capacitor $C_{1}$ is zero, the switch is closed. If $I_{0}$ be the current through inductor at $t=0$ , then for $t>0$ (initially $C_{2}$ is uncharged)

• A. maximum current through inductor equals $\frac{I_{0}}{2}$
• B. maximum current through inductor equals $\frac{C_{1} I_{0}}{C_{1} + C_{2}}$
• C. maximum charge on $C_{1}=\frac{C_{1} I_{0} \sqrt{L C_{2}}}{C_{1} + C_{2}}$
• D. maximum charge on $C_{1}=C_{1}I_{0}\sqrt{\frac{L}{C_{1} + C_{2}}}$

Answer 1

Answer: (D)

The maximum current through the inductor is $I_{0}$ .
By conservation of energy,
$\frac{1}{2}LI_{0}^{2}=\frac{1}{2}\left(C_{1} + C_{2}\right)V^{2}$
$V=\sqrt{\frac{L}{\left(C_{1} + C_{2}\right)}}I_{0}$
$Q_{1}=C_{1}V=C_{1}I_{0}\sqrt{\frac{L}{C_{1} + C_{2}}}$