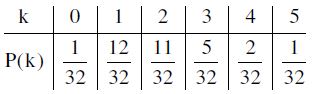Q. An unbiased coin is tossed 5 times. Suppose that a variable X is assigned the value k when k consecutive heads are obtained for k = 3, 4, 5, otherwise X takes the value — 1. Then the expected value of X, is :
Solution:
Expected value $ = \sum \,XP\left(k\right)$
$-\frac{1}{32}-\frac{12}{32}-\frac{11}{32}+\frac{15}{32}+\frac{8}{32}+\frac{5}{32}$
$= \frac{28-24}{32} = \frac{4}{32} = \frac{1}{8}$