Q.
An equilateral prism of mass $m$ rests on a rough horizontal surface with coefficient of friction $\mu$. A horizontal force $F$ is applied on the prism as shown in the figure. If the coefficient of friction is sufficiently high so that the prism does not slide before toppling, then the minimum force required to topple the prism is $\frac{m g}{\sqrt{n}}$. Find $n$.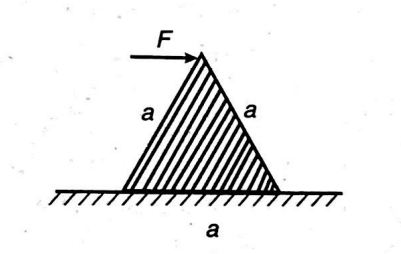
System of Particles and Rotational Motion
Solution: