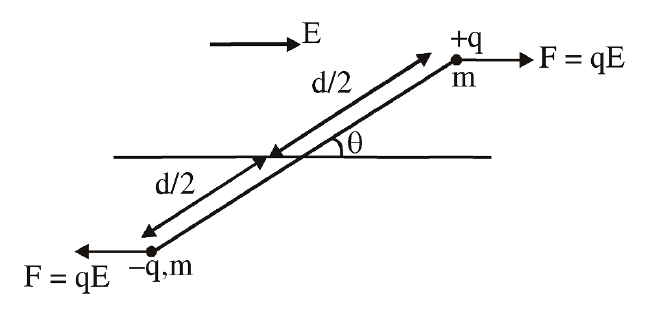
Q. An electric dipole is formed by two equal and opposite charges $q$ with separation $d$. The charges have same mass $m$. It is kept in a uniform electric field $E$. If it is slightly rotated from its equilibrium orientation, then its angular frequency $\omega$ is :
Solution:
moment of inertia (I) $ = m\left(\frac{d}{2}\right)^{2} \times2 = \frac{md^{2}}{2} $
Now by $\tau =I\alpha$
$ \left(qE\right) \left(d \sin\theta\right) = \frac{md^{2}}{2}.\alpha $
$ \alpha = \left(\frac{2qE}{md}\right)\sin\theta $
for small $\theta$
$ \Rightarrow \alpha = \left(\frac{2qE}{md}\right)\theta$
$ \Rightarrow $ Angular frequency $ \omega = \sqrt{\frac{2qE}{md}}$