Q.
A vertical spring mass system has the same time period as simple pendulum undergoing small oscillations. Now, both of them are put in an elevator going downwards with an acceleration $5 \,m / s ^{2}$. The ratio of time period of the spring mass system to the time period of the pendulum is (Assume, acceleration due to gravity, $g=10 \,m / s ^{2}$ )
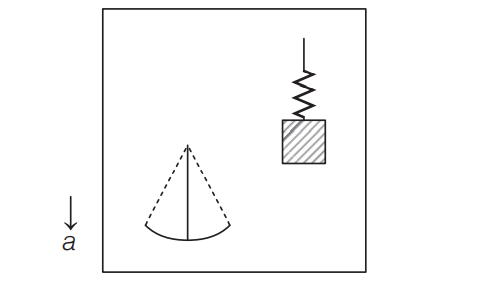
TS EAMCET 2019
Solution: