Q.
A uniform rod $A B$ of length $\ell$ and mass $m$ is free to rotate about point $A .$ The rod is released from rest in the horizontal position. Given that the moment of inertia of the rod about $A$ is $\frac{m \ell^{2}}{3}$, the initial angular acceleration of the rod will be:-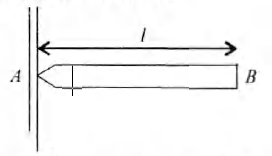
AIPMTAIPMT 2007System of Particles and Rotational Motion
Solution: