Question

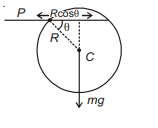
A uniform disc of mass $m$ and radius $R$ is pivoted at point $P$ and is free to rotate in vertical plane. The centre $C$ of disc is initially in horizontal position with $P$ as shown in figure. If it is released from this position, then its angular acceleration when the line $PC$ is inclined to the horizontal at an angle $\theta$ is

• A. $\frac{2 g \cos \theta}{3 R}$
• B. $\frac{g \sin \theta}{2 R}$
• C. $\frac{2 g \sin \theta}{R}$
• D. $\frac{2 g \sin \theta}{3 R}$

Answer 1

Answer: (A)

$\tau=I \alpha$
$m g(R \cos \theta)=\frac{3}{2} m r^{2} \alpha$
$\alpha=\frac{2 g \cos \theta}{3 r}$