Question

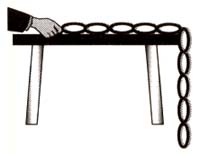
A uniform chain of length $16\,m$ and mass $540\,g$ whose $\frac{2}{3^{rd}}$ part of its length is hanging vertically down over the edge of the table and remaining part on the smooth table. Consider $g$ is $10\,ms^{- 2}$ , then the work required to pull the hanging part on to the table is $\frac{x}{10}J$ . Find $x$ .

Answer 1

The length of hanging part is $\frac{2 L}{3}$ , so its mass would be $\frac{2 M}{3}$ and will act at the centre of gravity G of hanging part, i.e., at a distance $\frac{L}{3}$ below the surface of table.
$\therefore $ Work done in pulling the hanging part on the
table $=$ increase in P.E.
$=mgh$
$=\frac{2 M}{3}\times g\times \frac{L}{3}$
$=\frac{2 MgL}{9}=\frac{2 \times 0 . 54 \times 10 \times 16}{9}$
Alternate Method
$F=\frac{M}{L}\left(\right.x\left.\right)g$
$\therefore W=Fdx=\frac{M}{L}\left(\right.x\left.\right)gdx$
$\therefore $ Total work done to pull the $\frac{2^{\text{rd }}}{3}$ part on the table,
$W=\int \limits_{0}^{\frac{2 L}{3}}\frac{M}{L}gxdx=\frac{M}{L}g\int\limits _{0}^{\frac{2 L}{3}}xdx$
$=\frac{M}{L}g\left[\frac{x^{2}}{2}\right]_{0}^{\frac{L}{3}}=\frac{M}{L}g\left[\frac{\left(\frac{2 L}{3}\right)^{2}}{2}\right]$
$=\frac{Mg}{L}\times \frac{4 L^{2}}{18}$
$\therefore W=\frac{2 M g L}{9}$
$\therefore W=\frac{2 \times 0 . 54 \times 10 \times 16}{9}$
$\therefore W=\frac{192}{10}J$
So, $x=192$ .