Q.
A uniform but time-varying magnetic field $B ( t )$ exists in a cylindrical region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point $P$ at a distance $r$ from the centre of the circular region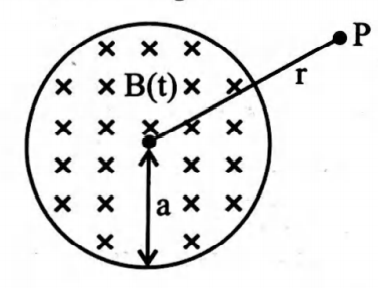
Electromagnetic Induction
Solution:
