Q.
A thin uniform bar of length $L$ and mass $8m$ lies on a smooth horizontal table. Two point masses $m$ and $2m$ are moving in the same horizontal plane from opposite sides of the bar with speeds $2v$ and $v$ respectively. The masses stick to the bar after collision at a distance $\frac{L}{3}$ and $\frac{L}{6}$ respectively from the centre of the bar. If the bar starts rotating about its center of mass as a result of collision, the angular speed of the bar will be :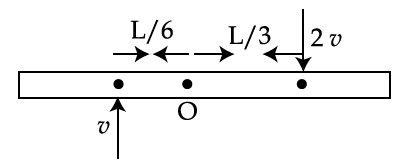
Solution: