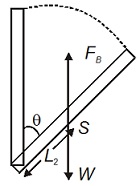
Question

A thin cylindrical rod $PQ$ of length $L$ and density $d_{1}$ is pivoted at its lowest point $\text{P}$ , inside a stationary homogeneous and non-viscous liquid of density $d_{2}$ . The rod is always fully submerged inside the liquid and is free to rotate in a vertical plane about a horizontal axis passing through $P$ . If $d_{1} < d_{2}$ , then the time period of small angular oscillations of the rod about its vertical equilibrium position will be

• A. $T=2\pi \sqrt{\frac{2 L}{3 g} \left(\frac{d_{1}}{d_{2} - d_{1}}\right)}$
• B. $T=2\pi \sqrt{\frac{L}{3 g} \left(\frac{d_{2}}{d_{2} - d_{1}}\right)}$
• C. $T=2\pi \sqrt{\frac{2 L}{g} \left(\frac{d_{1}}{d_{2} - d_{1}}\right)}$
• D. $T=2\pi \sqrt{\frac{2 L}{3 g} \left(\frac{d_{2} - d_{1}}{d_{1}}\right)}$

Answer 1

Answer: (A)

Consider the diagram in a displaced position.

The weight and upthrust $F_{B}$ , both pass through the centre of gravity $G$
$W=\left(\pi r^{2} L\right) \, d_{1}g$
$F_{B}=\left(\pi r^{2} L\right)d_{2}g$
The restoring torque about the pivot is
$\tau=-\left(\pi r^{2} L g \left(d_{2} - d_{1}\right) \frac{L}{2}\right)\theta $
$\alpha =-\frac{\left[\pi r^{2} L g \left(d_{2} - d_{1}\right) \frac{L}{2}\right] \theta }{\left(\left(\pi r^{2} L d_{1}\right) \, L^{2}\right)/3}$
$\alpha =-\left[\frac{3 g}{2 L} \left(\frac{d_{2} - d_{1}}{d_{1}}\right)\right]\theta $
$T=2\pi \sqrt{\frac{2 L}{3 g} \left(\frac{d_{1}}{d_{2} - d_{1}}\right)}$