Q.
A tennis ball (treated as hollow spherical shell) starting from $O$ rolls down a hill. At point A the ball becomes air borne leaving at an angle of $30^\circ$ with the horizontal. The ball strikes the ground at $B$. What is the value of the distance $AB$ ?
(Moment of inertia of a spherical shell of mass $m$ and radius $R$ about its diameter $=\frac{2}{3}mR^{2}$ )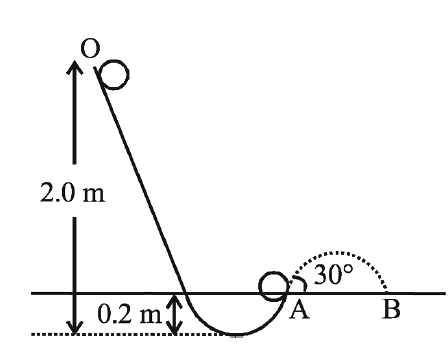
Solution: