Q.
A system of two identical, uniform discs with identical circular cavities, is shown in the figure. Different relevant coordinates are given in the figure. The coordinates of the centre of mass of the system are
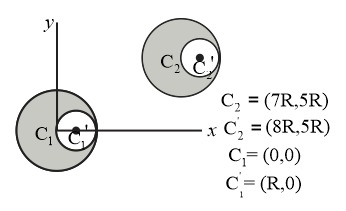
NTA AbhyasNTA Abhyas 2020
Solution: