Q.
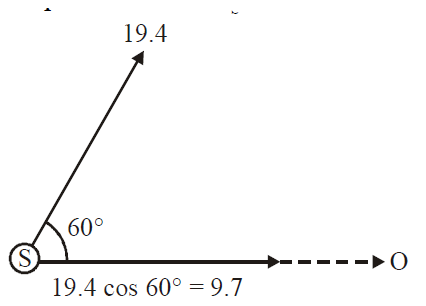
A source of sound $S$ emitting waves of frequency $100\, Hz$ and an observor $O$ are located at some distance from each other. The source is moving with a speed of $19.4\, m s^{-1}$ at an angle of $60^{\circ}$ with the source observer line as shown in the figure. The observor is at rest. The apparent frequency observed by the observer is (velocity of sound in air $330\, ms ^{-1}$ )
BITSATBITSAT 2016
Solution:
Here, original frequency of sound, $f_{0}=100 \,Hz$
Speed of source $V_{s} 19.4 \cos 60^{\circ}=9.7$
From Doppler's formula
$f^{1}=f_{0}\left(\frac{V-V_0}{V-V_{s}}\right)$
$f^{1}=100\left(\frac{V-0}{V-(+9.7)}\right)$
$f^{1} 100 \frac{V}{V\left(1-\frac{9.7}{V}\right)}$ $f^{1}=100\left(1+\frac{9.7}{330}\right)=103 \,Hz$
Apparent frequency $f^{1}=103 \,Hz$