Question

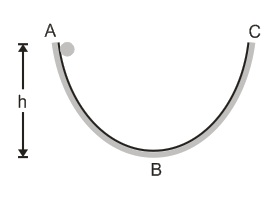
A solid ball rolls down a parabolic path $ABC$ from a height $h$ as shown in the figure. The portion $AB$ of the path is rough while $BC$ is smooth. How high will the ball climb in $BC$ ?

• A. $ H = \frac{5}{7} h ⁡$
• B. $H=\frac{5}{2} h$
• C. $H=\frac{7}{5} h$
• D. $H=\frac{3}{7} h$

Answer 1

Answer: (A)

Using the conservation of mechanical energy between $A$ and $B$
At $B$, total kinetic energy $=m g h$
Here, $m=$ mass of the ball
The ratio of rotational to translational kinetic energy would be,
$\frac{K_{R}}{K_{T}}=\frac{2}{5}$
$\therefore K_{R}=\frac{2}{7} m g h $
and $ K_{T}=\frac{5}{7} m g h$
In portion $B C$, friction is absent.
Therefore, rotational kinetic energy will remain constant and translational kinetic energy will convert into potential energy.
Hence, if $H$ be the height to which ball climbs in $B C$, then
$m g H=K_{T}$
or $m g H=\frac{5}{7} m g h$ or $ H=\frac{5}{7} h$