Question

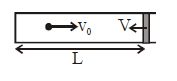
A small particle of mass m moving inside a heavy, hollow and straight tube along the tube axis undergoes elastic collision at two ends. The tube has no friction and it is closed at one end by a flat surface while the other end is fitted with a heavy movable flat piston as shown in figure. When the distance of the piston from closed end is L = L₀ the particle speed is v = v₀. The piston is moved inward at a very low speed V such that V << $\frac{dL}{L}$ V₀ , where dL is the infinitesimal displacement of the piston. Which of the following statement(s) is/are correct ?

• A. The rate at which the particle strikes the piston is v/L
• B. After each collision with the piston, the particle speed increases by 2V
• C. The particle's kinetic energy increases by a factor of 4 when the piston is moved inward from L₀ to $\frac{1}{2}$ L₀
• D. If the piston moves inward by dL, the particle speed increases by 2v $\frac{dL}{L}$

Answer 1

Answer: (B), (C)

(1) average rate of collision = $\frac{2L}{v}$

(2) speed of particle after collision $= 2V + v_{0}$
change in speed $= \left(2V + v_{0}\right) - v_{0}$
after each collision = 2V

no. of collision per unit time (frequency) $= \frac{v}{2L}$
change in speed in dt time $= 2V ×$ number of collision in dt time

$\Rightarrow \,dv = 2V \left(\frac{v}{2L}\right). \frac{dL}{V}$

$dv = \frac{vdL}{L}$

Now, $dv = - \frac{vdL}{L}$ {as L decrease}
$\int\limits^{v}_{v_0} \frac{dv}{v} = - \int\limits^{L_{0}/2}_{L_0} \frac{dL}{L}$

$\Rightarrow \,\left[ln \,v\right]^{v}_{v_0} = -\left[ln\,L\right]^{L_{0}/2}$
$\Rightarrow \,v = 2v_{0}$

$\Rightarrow \,KE_{L_0} = \frac{1}{2}mv^{2}_{0}\quad\quad \frac{KE_{L_0/2}}{KE_{0}} = 4$

$KE_{L_0/2} = \frac{1}{2}m\left(2v_{0}\right)^{2}$
or

$\left(dt\right)\left(\frac{v}{2x}\right) \frac{2mv}{dt} = F$

$F = \frac{mv^{2}}{x}$

$-m\,v \frac{dv}{dx} = \frac{mv^{2}}{x}$

$- \frac{dv}{v} = \frac{dx}{x}$

$ln \frac{v_{2}}{v_{1}} =ln \frac{x_{1}}{x_{2}}$
vx = constant $\Rightarrow $ on decreasing length to half K.E. becomes 1/4
$vdx + xdv = 0$