Q.
A small body of mass m slides down from the top A of a. smooth hill of height H with initial velocity zero. The hill becomes horizontal at the edge B whose height is h from the ground. Assume that the body falls at maximum distance away from the foot of the hill. Then the value of h is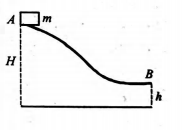
Work, Energy and Power
Solution: