Question

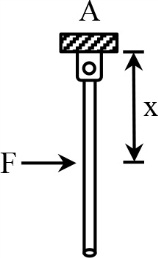
A rod of mass $m$ and length $l$ is hinged at one of its end $A$ as shown in figure. A force $F$ is applied at a distance $x$ from $A$ . The acceleration of the centre of mass $\left(a\right)$ varies with $x$ as -

• A.
• B.
• C.
• D.

Answer 1

Answer: (B)

The rod will rotate about point $A$ with angular acceleration:
$\alpha =\frac{\tau}{I}=\frac{F x}{\frac{m l^{2}}{3}}=\frac{3 F x}{m l^{2}}$
$\therefore a \, =\frac{l}{2} \, \alpha =\frac{3}{2}\frac{F x}{m l}$
or $a \, \propto \, x$
i.e., $a\text{-}x$ graph is a straight line passing through the origin.