Question

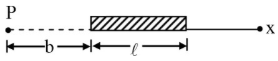
A rod of length $l$ is placed along x-axis. One of its ends is at the origin. The rod has a non-uniform charge density $\lambda =\frac{a}{x}$ , a being a positive constant. The electric potential at the point $P$ (origin) as shown in the figure is

• A. $V=\frac{a}{4 \pi \varepsilon_0}\left(\frac{l}{b(b+l)}\right)$
• B. $V=\frac{a}{4 \pi \varepsilon_0}\left(\frac{b}{l(b+l)}\right)$
• C. $V=\frac{a}{4 \pi \varepsilon_0}\left(\frac{b}{l}\right)$
• D. $V=\frac{a}{4 \pi \varepsilon_0}\left(\frac{l}{b}\right)$

Answer 1

Answer: (A)

$V \, =\displaystyle \int _{b}^{b + l}\frac{k d q}{x}=\displaystyle \int _{b}^{b + l}\frac{k \lambda d x}{x}= \, ka \, \displaystyle \int _{b}^{b + l}\frac{d x}{x^{2}}$
$V=ka \, \left(\frac{1}{b} - \frac{1}{b + l}\right)$
$V=\frac{a}{4 \pi \varepsilon_0}\left(\frac{l}{b(b+l)}\right)$