Q.
A rod of height $h$ is placed in a beaker of same radius. The height of the beaker is thrice its radius. An observer sees the
top end of the rod through a pin-hole (see figure). When the beaker is filled with a liquid (R.I. $=\sqrt{\mu}$ ) upto a height $2 h$, he can see the lower end of the rod. Find the value of $\mu$.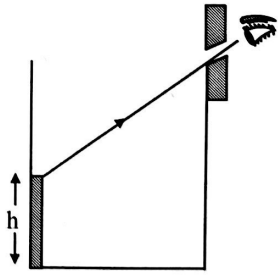
Ray Optics and Optical Instruments
Solution:
