Question

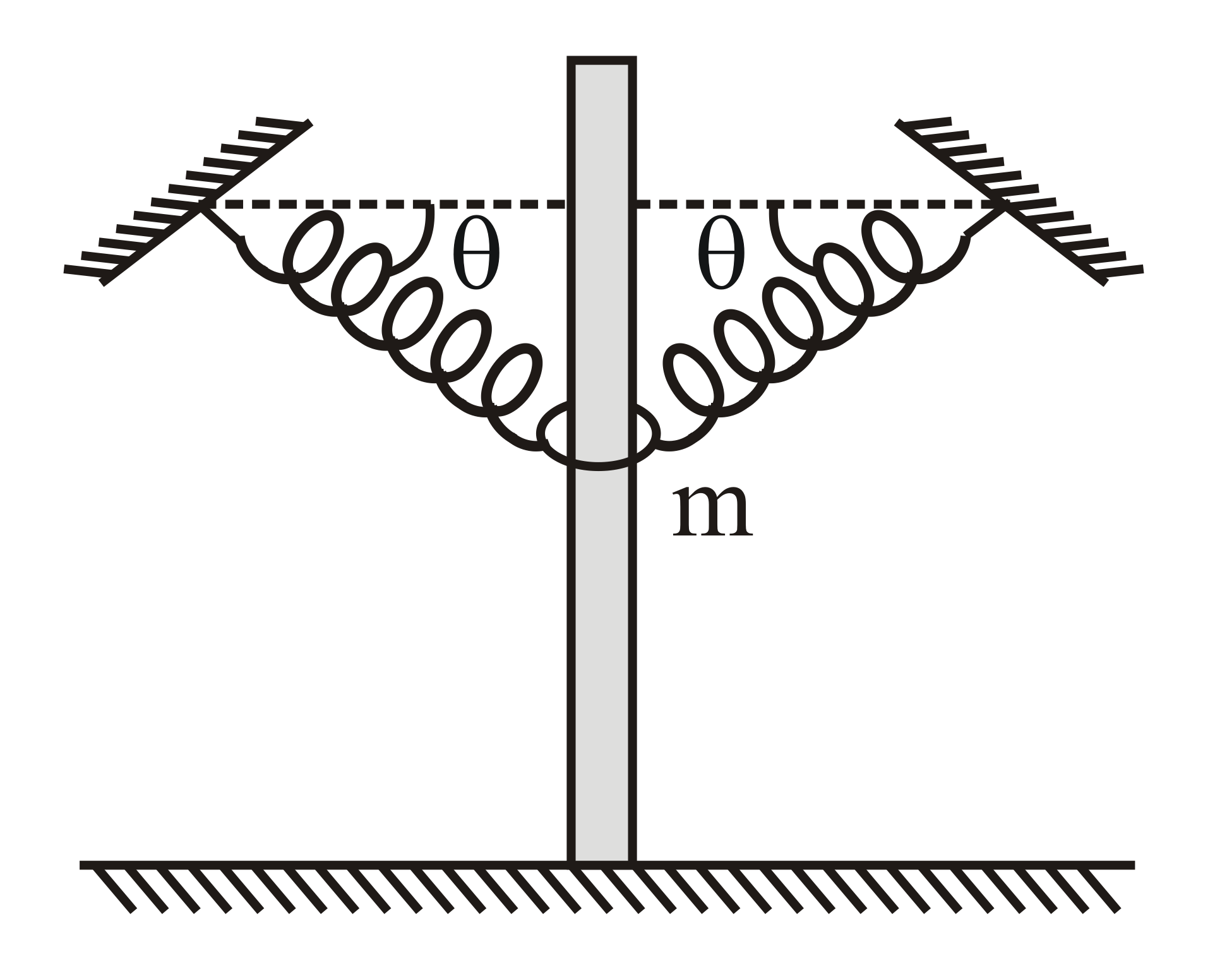
A ring of mass $m$ can freely slide on a smooth vertical rod. The ring is symmetrically attached with two springs (as shown in the figure) each of spring constant $k$ . Each spring makes an angle $\theta $ with the horizontal. What will be the time period of the ring if it is slightly displaced vertically?

• A. $2\pi \sqrt{\frac{m}{2 k}}$
• B. $2\pi \sqrt{\frac{m}{k}}$
• C. $2\pi \sqrt{\frac{2 m}{k sin^{2} \theta }}$
• D. $2\pi \sqrt{\frac{m}{2 k sin^{2} \theta }}$

Answer 1

Answer: (D)

If ring is pulled below the current position by displacement $y$ then elongation in the spring will be $x$ (for small displacement).
$y=xsin\theta $ ...(1)
If ring is displaced along $y$ ,
$ma=2kysin\theta $
$\Rightarrow ma=2kxsin^{2}\theta $
$\Rightarrow a=\left(\frac{2 k \left(sin\right)^{2} \theta }{m}\right)x$
$\Rightarrow \left(\omega \right)^{2}=\left(\frac{2 k \left(sin\right)^{2} \theta }{m}\right)$
Therefore, $T=2\pi \sqrt{\frac{m}{2 k sin^{2} \theta }}$