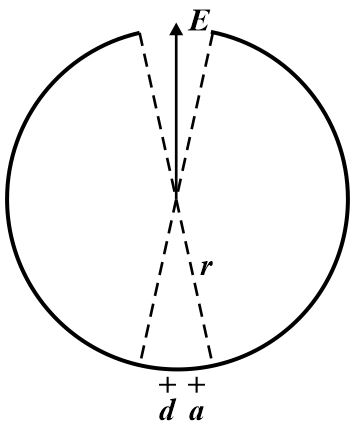
Question

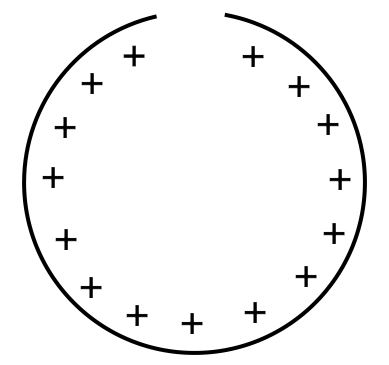
A ring of charge with radius $0.5 \, m$ has $0.002\pi \, m$ gap. If the ring carries a charge of $+1 C,$ the electric field at the centre is

• A. $7.5\times 10^{7} \, N \, C^{- 1}$
• B. $7.2\times 10^{7} \, N \, C^{- 1}$
• C. $6.2\times 10^{7} \, N \, C^{- 1}$
• D. $6.5\times 10^{7} \, N \, C^{- 1}$

Answer 1

Answer: (B)

Approaximate charge on the element opposite to the gap is
$\Delta q=\frac{Q}{2 \pi r}\left(0.002 \pi \right)$
$=\frac{1}{2 \pi \left(0.5\right)}\times \frac{2 \pi }{1000}=2\times \left(10\right)^{- 3 \, }C$
$E=\frac{9 \times 10^9 \times 2 \times 10^{-3}}{(0.5)^2}=7.2 \times 10^7 \mathrm{~N} \mathrm{C}^{-1}$